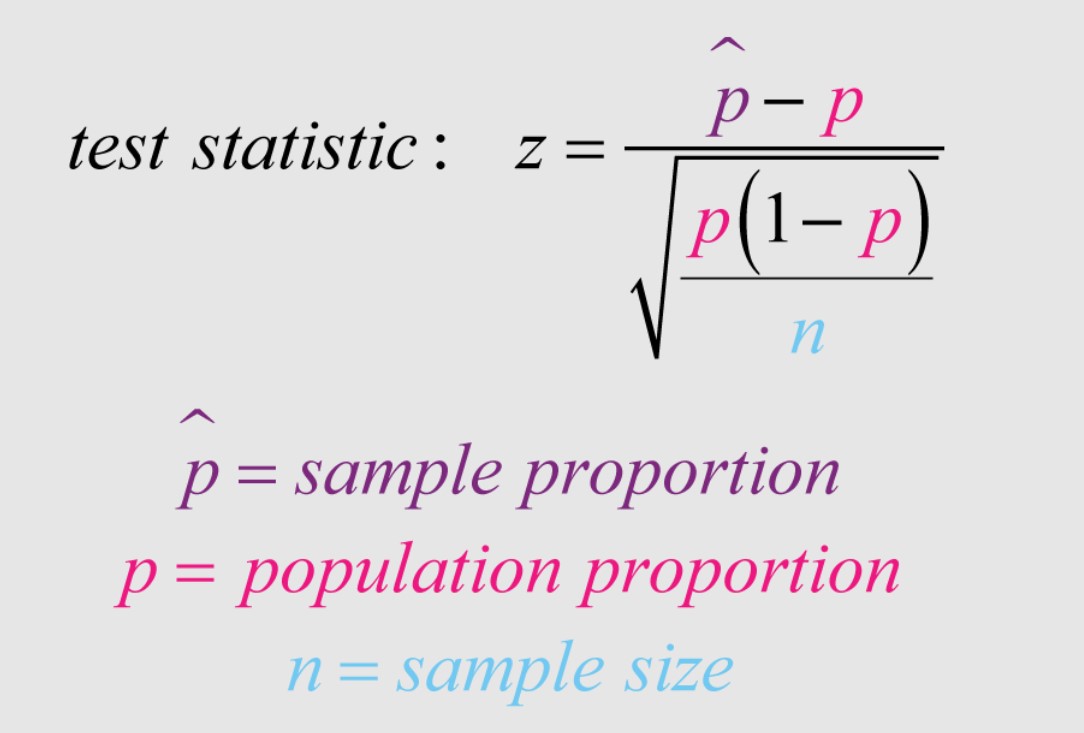
3 Easy Steps to Calculate Proportion in Statistics: Demystified!
Calculating proportion in statistics may seem daunting, but with our foolproof guide, you’ll be a pro in no time! 😊 Let’s dive right in and simplify this seemingly complex task!
Featured Image:
Source londongokestevenson.blogspot.com
Step 1: Define Your Population and Sample
The first step is to identify the population you’re interested in and then select a sample that represents that population. For example, if you want to know the proportion of people who prefer chocolate ice cream, your population would be all people, and your sample could be a group of 100 people randomly selected from that population.
Step 2: Count the Number of Successes
Next, count the number of successes in your sample. In our chocolate ice cream example, a success would be someone who prefers chocolate ice cream. Let’s say that you find that 60 out of the 100 people in your sample prefer chocolate ice cream.
Step 3: Calculate the Proportion
Finally, calculate the proportion by dividing the number of successes by the total sample size. In our example, the proportion of people who prefer chocolate ice cream is 60/100 = 0.6, or 60%.
Example:
Let’s say you want to know the proportion of students in your school who play a musical instrument. You randomly select 50 students and find that 25 of them play an instrument. Using the steps above, you can calculate the proportion as follows:
- Population: All students in your school
- Sample: 50 students randomly selected from your school
- Number of successes: 25 students who play an instrument
- Proportion: 25/50 = 0.5, or 50%
Comparison Table: Calculating Proportion
| Method | Calculation |
|---|---|
| Proportion | Number of successes / Total sample size |
| Percentage | Proportion x 100% |
| Ratio | Number of successes : Number of failures |
Additional Tips for Calculating Proportion
- Use a calculator: A calculator can make the process of calculating proportion much easier.
- Round your answer: When you calculate proportion, you will often get a decimal answer. Round your answer to the nearest hundredth or thousandth, depending on the level of accuracy you need.
- Be careful of sampling error: Sampling error is the difference between the proportion you calculate from your sample and the true proportion in the population. The larger your sample size, the smaller the sampling error will be.
Conclusion
Calculating proportion in statistics is a straightforward process that can be used to answer a variety of questions about a population. By following the steps outlined in this guide, you can calculate proportion with confidence! 😊
We hope you found this guide helpful! If you have any other questions about statistics, be sure to check out our other articles. 🎉
FAQ about Proportion in Statistics
Q: What is proportion?
A: Proportion is a measure of the number of occurrences of an event divided by the total number of possible outcomes.
Q: How do I calculate proportion?
A: Proportion = Number of occurrences / Total number of outcomes
Q: What is the difference between proportion and percentage?
A: Proportion is a value between 0 and 1, while percentage is a value between 0% and 100%. Proportion is a raw value, while percentage is a scaled version of proportion.
Q: How do I calculate the proportion of success?
A: Proportion of success = Number of successes / Total number of trials
Q: How do I interpret the proportion?
A: The proportion represents the likelihood of an event occurring. A higher proportion indicates a higher likelihood.
Q: How can I use proportion in research?
A: Proportion can be used to compare different groups, assess the effectiveness of interventions, and draw conclusions about the population.
Q: What is a confidence interval for a proportion?
A: A confidence interval is a range of values that is likely to contain the true proportion. It is used to estimate the precision of the proportion estimate.
Q: How do I calculate the confidence interval for a proportion?
A: Confidence interval = Proportion ± Margin of error
- Margin of error = t-value * (Square root of (Proportion * (1-Proportion) / Sample size))
Q: What is the sample size required for a proportion estimate?
A: The sample size required depends on the desired margin of error and the expected proportion.
Q: How do I avoid bias in my proportion estimate?
A: To avoid bias, ensure that the sample is representative of the population and that there are no confounding factors.